Staff profile
Professor David Tozer
Professor

| Affiliation | Telephone |
|---|---|
| Professor in the Department of Chemistry | +44 (0) 191 33 42111 |
Biography
Research Interests
Electronic structure calculations are becoming an integral part of chemistry research, where they play a vital role in complementing and aiding interpretation of experimental data. To be useful, a method must provide an accurate description of the ground state of a molecule or solid, together with its response to structural, electric and magnetic perturbations. By far the most widely used electronic structure method is Kohn-Sham density functional theory (DFT), whose modest computational cost makes it applicable to large, chemically, physically and biologically relevant systems. The aim of our research is to improve the quality of DFT predictions, particularly in areas where the method is currently deficient. We collaborate with a number of international research groups, notably Helgaker (Oslo, Norway), De Proft and Geerlings (Brussels, Belgium), Ruud (Tromso, Norway), Cohen (Cambridge, UK), O'Hagan (St Andrews, UK) and Williams (Durham, UK).
Current interests include:
Exchange–Correlation Functionals
The main challenge in DFT is the accurate description of the non-classical exchange and correlation interactions between electrons. A central theme of our research is the development of improved approximations to this exchange–correlation energy. Our B97-2 approximation is a high-quality all-round functional, which has been implemented in all major commercial electronic structure programs, including Gaussian. Our KT1, KT2 and KT3 approximations were designed specifically to provide high quality NMR shielding constants and chemical shifts. Presently, we are investigating two approaces to the exchange–correlation problem. First, we are investigating Coulomb attenuated functionals, which split the Coulomb interaction into short- and long-range components. See here for further details.
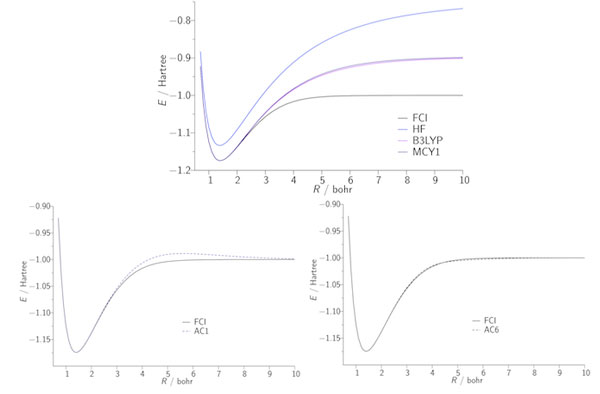
Our second approach to the exchange–correlation problem involves the adiabatic connection, which expresses the exchange–correlation energy as an integral over the electron–electron coupling strength. As an illustration, the figures below present potential energy curves of the H2 molecule; the FCI curve is exact. B3LYP and MCY are existing approximations, which fail to dissociate the molecule correctly due to the lack of static correlation. The AC1 and AC6 curves quantify what can be achieved using a simple adiabatic connection form with exact input data.
Theoretical Electronic Spectroscopy
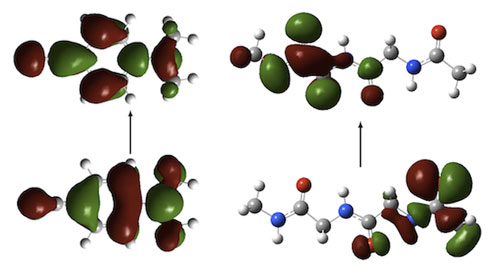
It is well-established that DFT electronic excitation energies to charge-transfer (CT) states-for example in the dipeptide below right-are often significantly underestimated when GGA and hybrid functionals are used, whilst others such as the CT state in DMABN (left) are accurately described.
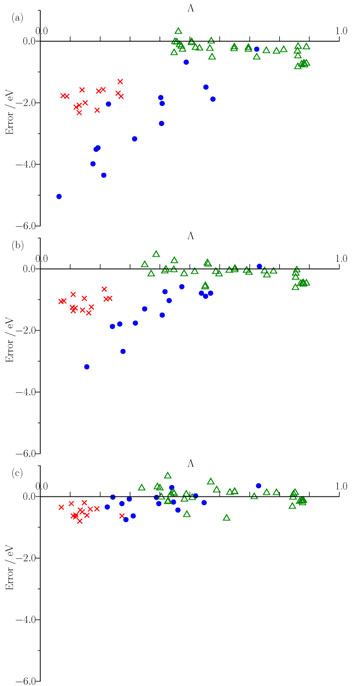
We have provided insight into this observation through a consideration of the extent to which excitation energy errors correlate with the degree of spatial overlap between the occupied and virtual orbitals involved in the excitations. The figure below plots excitation energy errors as a function of the degree of orbital overlap, Λ, for three functionals, (a) the PBE GGA, (b) the B3LYP hybrid, and (c) the CAM-B3LYP Coulomb attenuated approximation. Each point corresponds to a single excitation, with local exciations in green, Rydberg excitations in red, and CT excitations in blue. The results highlight the good quality predictions from CAM-B3LYP. For GGA and hybrid, there is a clear correlation between error and overlap, allowing a diagnostic test to be proposed. See here for full details. We have also discussed the origin of the failure in terms of the integer discontinuity in model systems.
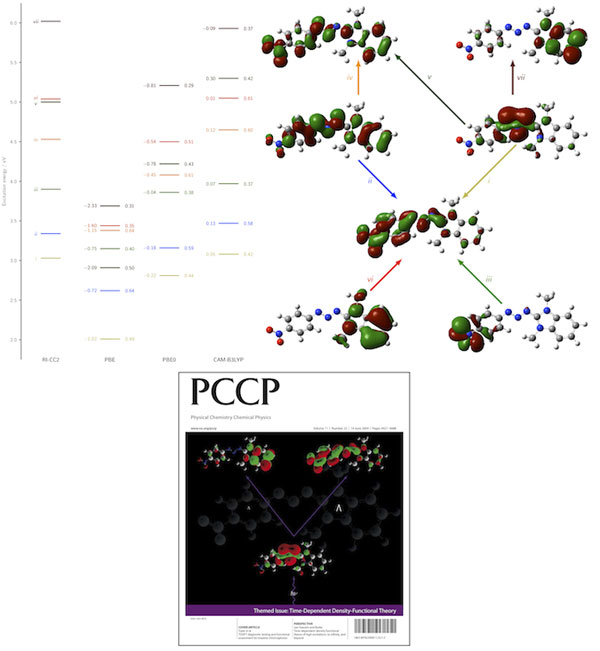
As a pertinent application of the diagonstic method and a further demonstration of the success of the CAM-B3LYP functional in describing excited states in organic molecules, we applied both to the triazene system shown below. The orbitals which represent the electronic excitations (i)-(vii) are shown, together with the excitation energy error (relative to RI-CC2) and Λ values for each transition. The success of CAM-B3LYP is evident, as is the low-overlap failure of the PBE GGA. Click the PCCP cover below for full details.
We have also applied Coulomb-attenuated functionals to the electronic (and structural) properties of polyacetylene and polyyne.
In our most recent work we have considered the implications of low overlap in excited state surfaces. For common density functional approximations, the accuracy of the surface will be non-uniform if the spatial overlap between the occupied and virtual orbitals involved in the excitation has a strong conformational dependence; the excited state surface will collapse toward the ground state in regions where the overlap is very low. This is illustrated below for DMABN. For both the LE and ICT excitations, the overlap drops very low upon twisting and so both states collapse with PBE and to a lesser extent B3LYP. This breakdown is eliminated using CAM-B3LYP. See here for full details.

Magnetic Response Parameters; Kohn–Sham Equations; Optimised Effective Potentials
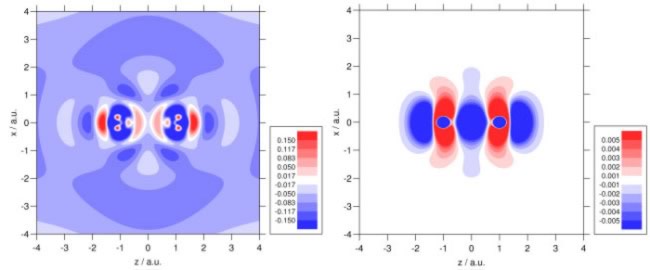
For hybrid functionals, we have demonstrated that the quality of magnetic response parameters is highly sensitive to the formulation of the Kohn-Sham equations - significant improvements are obtained when the equations involve a well-defined multiplicative potential. Our investigations have considered the Wu-Yang/Zhao-Morrison-Parr approaches, together with the localised Hartree-Fock approximation (LHF) and the rigorous optimised effective potential approach (OEP); we have highlighted unexpected difference between the latter two methods. The figures below compare the LHF and OEP potentials and electron densities. See here for further details.
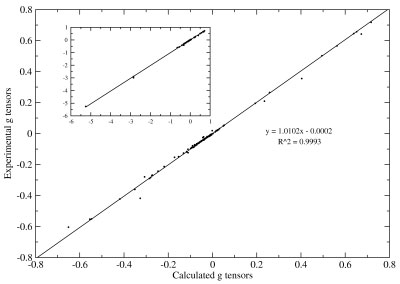
Recent studies have focused on transition metal NMR chemical shifts and rotational g tensors; the correlation plot below highlights the accuracy in the latter. Related properties of interest include indirect nuclear spin–spin coupling constants and NMR of the solid state.
Conceptual DFT; Studies of Temporary Anions
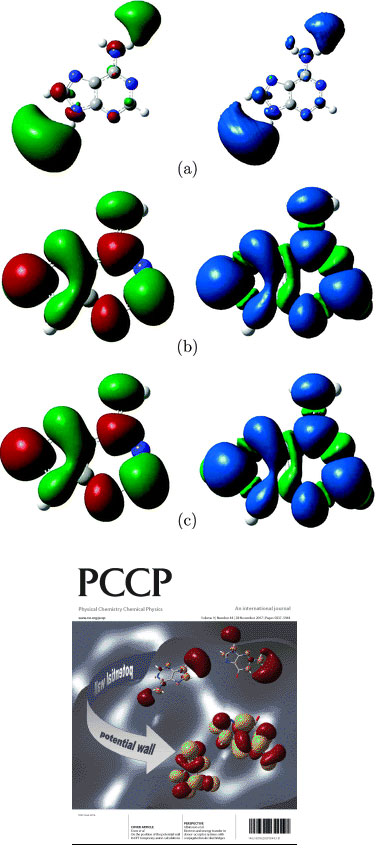
Conceptual DFT is concerned with the calculation of familiar chemical concepts such as hardness, softness, and electronegativity, from quantities such as the ionisation potential and electron affinity. We have developed a novel method for calculating negative electron affinities, which avoids an explicit calculation on the (problematic) temporary anion. The method yields results that correlate well with experimental values from electron transmission spectroscopy. Insight into the method is provided by an analysis of the integer discontinuity. We have recently extended this work to allow explicit DFT calculations on temporary anions. The left hand figure below shows the singly occupied molecular orbital (left) and spin density (right) in the adenine anion. Part (a) is a conventional DFT calculation with a diffuse basis set-the electron is clearly leaving the system. Parts (b) and (c) use our proposed approach with a potential wall and optimally compact set, respectively. Click on the PCCP cover below for full details.
Other recent work includes an investigation of confinement effects on chemical reactivity.
Insight from High Quality Electron Densities
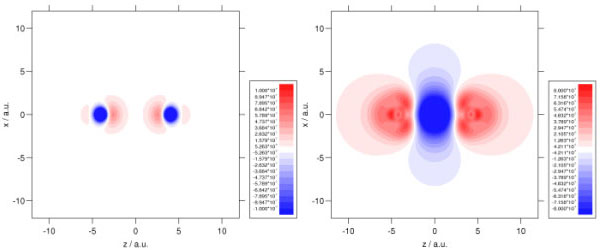
We make extensive use of high quality ab initio electron densities, in order to help us understand why DFT sometimes fails and hence improve the theory. For example, investigations demonstrated that an improved electron density could reduce DFT shielding constant errors by almost a factor of three. Ab initio densities also help us to understand why DFT fails to describe long-range der Waals interactions- it fails to distort the electron densities towards one another. The plots below show the density distortion and associated interaction correlation potential in the helium dimer, determined using coupled cluster theory; local DFT functionals cannot reproduce this behaviour! See here for further details. It is vital to resolve this deficiency if the full potential of DFT is to be realised in the field of intermolecular forces. We are also using high quality densities in our investigations of the adiabatic connection (see above).
Potential Energy Surfaces and Conformational Preferences
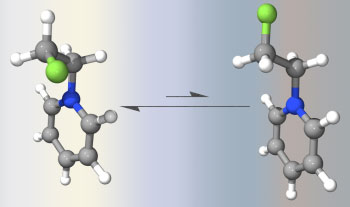
In addition to theory development, we apply DFT to real chemical and biological problems. We are particularly interested in the calculation of theoretically challenging potential energy surfaces (e.g. oxirene-ketene interconversion) and the study of conformational preferences in organofluorine chemistry, arising due to the gauche effect or intramolecular electrostatic interactions. Click on the figure below for another example.
Research interests
- Methodological Developments in Density Functional Theory
- Theoretical Spectroscopy
- Excited States
Publications
Chapter in book
- Conceptual DFT and ConfinementGeerlings, P., Tozer, D. J., & De Proft, F. (2021). Conceptual DFT and Confinement. In P. K. Chattaraj & D. Chakraborty (Eds.), Chemical Reactivity in Confined Systems: Theory, Modelling and Applications (pp. 49-68). Wiley.
Conference Paper
- Importance of the exchange-correlation potential in Kohn-Sham theoryTozer, D. (2006). Importance of the exchange-correlation potential in Kohn-Sham theory. In Abstracts of Papers of the American Chemical Society.
Journal Article
- Classical Reaction Barriers in DFT: An Adiabatic-Connection Perspective.Wibowo-Teale, A. M., Huynh, B. C., Helgaker, T., & Tozer, D. J. (2025). Classical Reaction Barriers in DFT: An Adiabatic-Connection Perspective. Journal of Chemical Theory and Computation, 21(1), 124-137. https://doi.org/10.1021/acs.jctc.4c01038
- On the Topological Phase around Conical Intersections with Tamm–Dancoff Linear-Response Time-Dependent Density Functional TheoryTaylor, J. T., Tozer, D. J., & Curchod, B. F. E. (2024). On the Topological Phase around Conical Intersections with Tamm–Dancoff Linear-Response Time-Dependent Density Functional Theory. The Journal of Physical Chemistry A, 128(27), 5314-5320. https://doi.org/10.1021/acs.jpca.4c02503
- Effective homogeneity of Fermi-Amaldi-containing exchange-correlation functionalsTozer, D. J. (2023). Effective homogeneity of Fermi-Amaldi-containing exchange-correlation functionals. The Journal of Chemical Physics, 159(24), Article 244102. https://doi.org/10.1063/5.0179111
- On the description of conical intersections between excited electronic states with LR-TDDFT and ADC(2).Taylor, J. T., Tozer, D. J., & Curchod, B. F. E. (2023). On the description of conical intersections between excited electronic states with LR-TDDFT and ADC(2). The Journal of Chemical Physics, 159(21), Article 214115. https://doi.org/10.1063/5.0176140
- DFT Exchange: Sharing Perspectives on the Workhorse of Quantum Chemistry and Materials ScienceTeale, A. M., Helgaker, T., Savin, A., Adano, C., Aradi, B., Arbuznikov, A. V., Ayers, P., Baerends, E. J., Barone, V., Calaminici, P., Cances, E., Carter, E. A., Chattaraj, P. K., Chermette, H., Ciofini, I., Crawford, T. D., De Proft, F., Dobson, J., Draxl, C., … Yang, W. (2022). DFT Exchange: Sharing Perspectives on the Workhorse of Quantum Chemistry and Materials Science. Physical Chemistry Chemical Physics, 24(47), 28700-28781. https://doi.org/10.1039/d2cp02827a
- Incorporation of the Fermi–Amaldi Term into Direct Energy Kohn–Sham CalculationsDillon, D. J., & Tozer, D. J. (2022). Incorporation of the Fermi–Amaldi Term into Direct Energy Kohn–Sham Calculations. Journal of Chemical Theory and Computation, 18(2), 703-709. https://doi.org/10.1021/acs.jctc.1c00840
- Thermodynamic equilibrium between locally excited and charge-transfer states through thermally activated charge transfer in 1-(pyren-2′-yl)-o-carboraneJi, L., Riese, S., Schmiedel, A., Holzapfel, M., Fest, M., Nitsch, J., Curchod, B. F., Friedrich, A., Wu, L., Al Mamari, H. H., Hammer, S., Pflaum, J., Fox, M. A., Tozer, D. J., Finze, M., Lambert, C., & Marder, T. B. (2022). Thermodynamic equilibrium between locally excited and charge-transfer states through thermally activated charge transfer in 1-(pyren-2′-yl)-o-carborane. Chemical Science, 13, 5205-5219. https://doi.org/10.1039/d1sc06867a
- New density-functional approximations and beyond: general discussionBrandenburg, J. G., Burke, K., Cancio, A., Erhard, J., Fromager, E., Ghosal, A., Gidopoulos, N., Gori-Giorgi, P., Helgaker, T., Hourahine, B., Jacob, C. R., Kooi, D., Maitra, N., Mulay, M. R., Pernal, K., Pribram-Jones, A., Reining, L., Romaniello, P., Ryder, M. R., … Yang, W. (2020). New density-functional approximations and beyond: general discussion. Faraday Discussions, 224, 166-200. https://doi.org/10.1039/d0fd90023k
- Low energy electron impact resonances of anthracene probed by 2D photoelectron imaging of its radical anionMensa-Bonsu, G., Lietard, A., Tozer, D. J., & Verlet, J. R. (2020). Low energy electron impact resonances of anthracene probed by 2D photoelectron imaging of its radical anion. The Journal of Chemical Physics, 152(17), Article 174303. https://doi.org/10.1063/5.0007470
- Photoelectron spectroscopy of para-benzoquinone cluster anionsMensa-Bonsu, G., Wilson, M. R., Tozer, D. J., & Verlet, J. R. (2019). Photoelectron spectroscopy of para-benzoquinone cluster anions. Journal of Chemical Physics, 151(20), Article 204302. https://doi.org/10.1063/1.5132391
- Photoelectron spectroscopic study of I−·ICF3: a frontside attack SN2 pre-reaction complexMensa-Bonsu, G., Tozer, D. J., & Verlet, J. R. (2019). Photoelectron spectroscopic study of I−·ICF3: a frontside attack SN2 pre-reaction complex. Physical Chemistry Chemical Physics, 21(26), 13977-13985. https://doi.org/10.1039/c8cp06593d
- Simple DFT Scheme for Estimating Negative Electron AffinitiesVibert, C. P., & Tozer, D. J. (2018). Simple DFT Scheme for Estimating Negative Electron Affinities. Journal of Chemical Theory and Computation, 15(1), 241-248. https://doi.org/10.1021/acs.jctc.8b00938
- Molecular excited states from the SCAN functionalTozer, D. J., & Peach, M. J. (2018). Molecular excited states from the SCAN functional. Molecular Physics, 116(11), 1504-1511. https://doi.org/10.1080/00268976.2018.1453094
- Approximating the Shifted Hartree-Exchange-Correlation Potential in Direct Energy Kohn–Sham TheorySharpe, D. J., Levy, M., & Tozer, D. J. (2018). Approximating the Shifted Hartree-Exchange-Correlation Potential in Direct Energy Kohn–Sham Theory. Journal of Chemical Theory and Computation, 14(2), 684-692. https://doi.org/10.1021/acs.jctc.7b01060
- Range-separation parameter in tuned exchange–correlation functionals: Successive ionizations and the Fukui functionGledhill, J. D., De Proft, F., & Tozer, D. J. (2016). Range-separation parameter in tuned exchange–correlation functionals: Successive ionizations and the Fukui function. Journal of Chemical Theory and Computation, 12(10), 4879-4884. https://doi.org/10.1021/acs.jctc.6b00709
- Nicholas Charles Handy. 17 June 1941 - 2 October 2012Clary, D., Knowles, P., & Tozer, D. (2015). Nicholas Charles Handy. 17 June 1941 - 2 October 2012. Biographical Memoirs of the Fellows of the Royal Society, 61, 145-160. https://doi.org/10.1098/rsbm.2015.0002
- Fractional electron loss in approximate DFT and Hartree-Fock theoryPeach, M., Teale, A., Helgaker, T., & Tozer, D. (2015). Fractional electron loss in approximate DFT and Hartree-Fock theory. Journal of Chemical Theory and Computation, 11(11), 5262-5268. https://doi.org/10.1021/acs.jctc.5b00804
- System-dependent exchange–correlation functional with exact asymptotic potential and εHOMO ≈ − IGledhill, J. D., & Tozer, D. J. (2015). System-dependent exchange–correlation functional with exact asymptotic potential and εHOMO ≈ − I. Journal of Chemical Physics, 143(2), Article 024104. https://doi.org/10.1063/1.4926397
- ForewordHelgaker, T., Knowles, P., Lee, T., Rice, J., & Tozer, D. (2015). Foreword. Molecular Physics, 113(13-14), 1509-1510. https://doi.org/10.1080/00268976.2015.1047162
- Molecular properties in the Tamm–Dancoff approximation: indirect nuclear spin–spin coupling constantsCheng, C., Ryley, M., Peach, M., Tozer, D., Helgaker, T., & Teale, A. (2015). Molecular properties in the Tamm–Dancoff approximation: indirect nuclear spin–spin coupling constants. Molecular Physics, 113(13-14), 1937-1951. https://doi.org/10.1080/00268976.2015.1024182
- Molecular Binding in Post-Kohn-Sham Orbital-Free DFTBorgoo, A., Green, J., & Tozer, D. (2014). Molecular Binding in Post-Kohn-Sham Orbital-Free DFT. Journal of Chemical Theory and Computation, 10(12), 5338-5345. https://doi.org/10.1021/ct500670h
- Revisiting the density scaling of the non-interacting kinetic energyBorgoo, A., Teale, A., & Tozer, D. (2014). Revisiting the density scaling of the non-interacting kinetic energy. Physical Chemistry Chemical Physics, 16(28), 14578-14583. https://doi.org/10.1039/c4cp00170b
- Density functional theory and its applicationsTozer, D. J., & Peach, M. J. (2014). Density functional theory and its applications. Physical Chemistry Chemical Physics, 16(28), 14333-14333. https://doi.org/10.1039/c4cp90074j
- Atomic electron affinities and the role of symmetry between electron addition and subtraction in a corrected Koopmans approachTeale, A., De Proft, F., Geerlings, P., & Tozer, D. (2014). Atomic electron affinities and the role of symmetry between electron addition and subtraction in a corrected Koopmans approach. Physical Chemistry Chemical Physics, 16(28), 14420-14434. https://doi.org/10.1039/c3cp54528h
- Assessment of tuning methods for enfacing approximate energy linearity in range-separated hybrid functionalsGledhill, J. D., Peach, M. J., & Tozer, D. J. (2013). Assessment of tuning methods for enfacing approximate energy linearity in range-separated hybrid functionals. Journal of Chemical Theory and Computation, 9(10), 4414-4420. https://doi.org/10.1021/ct400592a
- TDDFT diagnostic testing and functional assessment for triazene chromophoresPeach, M. J. G., Le Sueur, C. R., Ruud, K., Guillaume, M., & Tozer, D. J. (2009). TDDFT diagnostic testing and functional assessment for triazene chromophores. Physical Chemistry Chemical Physics, 11(22), 4465-4470. https://doi.org/10.1039/b822941d
- Choice of exchange-correlation functional for computing NMR indirect spin-spin coupling constants.Keal, T., Helgaker, T., Salek, P., & Tozer, D. (2006). Choice of exchange-correlation functional for computing NMR indirect spin-spin coupling constants. Chemical Physics Letters, 425(1-3), 163-166. https://doi.org/10.1016/j.cplett.2006.05.032
- Influence of Coulomb-attenuation on exchange-correlation functional quality.Peach, M., Cohen, A., & Tozer, D. (2006). Influence of Coulomb-attenuation on exchange-correlation functional quality. Physical Chemistry Chemical Physics, 8(39), 4543-4549. https://doi.org/10.1039/b608553a
- The intramolecular β-fluorine⋯ammonium interaction in 4- and 8-membered rings.Gooseman, N., O’Hagan, D., Slawin, A., Teale, A., Tozer, D., & Young, R. (2006). The intramolecular β-fluorine⋯ammonium interaction in 4- and 8-membered rings. Chemical Communications, 2006(30), 3190-3192. https://doi.org/10.1039/b606334a
- Assessment of a Coulomb-attenuated exchange-correlation energy functionalPeach, M., Helgaker, T., Salek, P., Keal, T., Lutnaes, O., Tozer, D., & Handy, N. (2005). Assessment of a Coulomb-attenuated exchange-correlation energy functional. Physical Chemistry Chemical Physics, 8(5), 558-562. https://doi.org/10.1039/b511865d
- Nonlocal density-functional description of exchange and correlation in siliconRushton, P., Tozer, D., & Clark, S. (2002). Nonlocal density-functional description of exchange and correlation in silicon. Physical Review B, 65(23), Article 235203. https://doi.org/10.1103/physrevb.65.235203
- Description of exchange and correlation in the strongly inhomogeneous electron gas using a nonlocal density functionalRushton, P., Tozer, D., & Clark, S. (2002). Description of exchange and correlation in the strongly inhomogeneous electron gas using a nonlocal density functional. Physical Review B, 65(19), Article 193106. https://doi.org/10.1103/physrevb.65.193106
- Density-functional calculations of semiconductor properties using a semiempirical exchange-correlation functionalRushton, P., Clark, S., & Tozer, D. (2001). Density-functional calculations of semiconductor properties using a semiempirical exchange-correlation functional. Physical Review B, 63(11), Article 115206. https://doi.org/10.1103/physrevb.63.115206
- Experimental assessment of lanthanide ion donor preference: spectroscopic and theoretical dissection of static charge and dynamic polarisation contributions to axial ligation in a C-4- symmetric chiral europium complexBruce, J., Parker, D., & Tozer, D. (2001). Experimental assessment of lanthanide ion donor preference: spectroscopic and theoretical dissection of static charge and dynamic polarisation contributions to axial ligation in a C-4- symmetric chiral europium complex. Chemical Communications, 21, 2250-2251.
- Correlation of optical and NMR spectral information with coordination variation for axially symmetric macrocyclic Eu(III) and Yb(III) complexes: axial donor polarisability determines ligand field and cation donor preferenceDickins, R., Parker, D., Bruce, J., & Tozer, D. (n.d.). Correlation of optical and NMR spectral information with coordination variation for axially symmetric macrocyclic Eu(III) and Yb(III) complexes: axial donor polarisability determines ligand field and cation donor preference. Dalton Transactions, 7, 1264-1271.

